LEAKED!: 100% REAL 2025 JAMB Mathematics QUESTIONS AND ANSWERS EXPO

Are looking for 100% REAL 2025 JAMB Mathematics questions and answers EXPO, if yes search no more, you are at the right page

Stay Updated:

Join our JAMB, WAEC, NECO, POST UTME, e.t.c Questions and Answers WhatsApp channel and Telegram channel for early expo, updates, and live practice sessions.

1. Question 7

• Question: Simplify (27²)⁻¹ᐟ³
  (A) 1/9
  (B) 1/18
  (C) 1/6
  (D) 1/3
• Topic: Laws of Indices (Exponents)
• Correct Answer: A
• Explanation:
  1. Apply the power of a power rule: (aᵐ)ⁿ = aᵐⁿ.
     (27²)⁻¹ᐟ³ = 27^(2 × -1/3) = 27⁻²/³
  2. Express the base (27) as a power of a prime number: 27 = 3³.
     So, 27⁻²/³ = (3³)⁻²/³
  3. Apply the power of a power rule again:
     (3³)⁻²/³ = 3^(3 × -2/3) = 3⁻²
  4. Apply the negative exponent rule: a⁻ⁿ = 1/aⁿ.
     3⁻² = 1/3² = 1/9
• Why other options are incorrect:
  • (B) 1/18: This might result from incorrectly calculating 3² as 6 and then maybe multiplying by 3? It doesn’t follow index laws.
  • (C) 1/6: This might arise from incorrectly treating the exponents or the base.
  • (D) 1/3: This would be 3⁻¹, potentially from ignoring the exponent ‘2’ inside the bracket or in the final step.

2. Question 9

• Question: Simplify: – log₁₀ 0.00001
  (A) -5
  (B) -4
  (C) 5
  (D) 4
• Topic: Logarithms
• Correct Answer: C
• Explanation:
  1. Express the number 0.00001 in standard form (power of 10): 0.00001 = 1/100000 = 1/10⁵ = 10⁻⁵.
  2. Substitute this into the logarithm: log₁₀ 0.00001 = log₁₀(10⁻⁵).
  3. Use the logarithm property log<0xE1><0xB5><0x83> bˣ = x: log₁₀(10⁻⁵) = -5.
  4. The original expression has a negative sign outside the logarithm: – (log₁₀ 0.00001) = – (-5).
  5. Simplify: -(-5) = 5.
• Why other options are incorrect:
  • (A) -5: This is the value of log₁₀ 0.00001, but the question asks for the negative of this value.
  • (B) -4: This would result from incorrectly identifying the power of 10 (e.g., counting zeros instead of decimal places correctly).
  • (D) 4: Incorrect power of 10 and forgetting the negative sign outside the log.

3. Question 8

• Question: Simplify 4log(x-2) – 2log(x-3)
  (A) log[ (x-2)⁴ / (x-3)² ](B) 5
  (C) 4
  (D) log[ (x-2)⁴ (x-3)² ]
• Topic: Laws of Logarithms
• Correct Answer: A
• Explanation:
  1. Apply the power rule of logarithms (n log a = log aⁿ):
     4log(x-2) = log(x-2)⁴
     2log(x-3) = log(x-3)²
  2. Substitute these back into the expression:
     log(x-2)⁴ – log(x-3)²
  3. Apply the subtraction rule of logarithms (log a – log b = log (a/b)):
     log(x-2)⁴ – log(x-3)² = log[ (x-2)⁴ / (x-3)² ]
• Why other options are incorrect:
  • (B) 5, (C) 4: The simplification of a logarithmic expression involving variables typically results in another logarithmic expression, not a constant, unless specific values of x lead to cancellations not generally applicable.
  • (D) log[ (x-2)⁴ (x-3)² ]: This would be the result if the original expression involved addition (log a + log b = log(ab)) instead of subtraction.

4. Question 3

• Question: Calculate the volume of a cube whose length is 3cm.
  (A) 9cm²
  (B) 9cm³
  (C) 27cm²
  (D) 27cm³
• Topic: Mensuration (Volume of Solids)
• Correct Answer: D
• Explanation:
  1. The formula for the volume (V) of a cube with side length (L) is V = L³.
  2. Given L = 3cm.
  3. Substitute the value: V = (3cm)³ = 3cm × 3cm × 3cm = 27 cm³.
• Why other options are incorrect:
  • (A) 9cm²: This calculates the area of one face (L² = 3² = 9) and uses area units (cm²), not volume units.
  • (B) 9cm³: This incorrectly calculates the volume as L² but uses the correct volume units.
  • (C) 27cm²: This has the correct numerical value for the volume but uses incorrect area units (cm²).

5. Question 2

• Question: The chord of a circle of radius 17cm is 30cm long. Calculate the distance of the chord from the centre of the circle.
  (A) 15 cm
  (B) 47 cm
  (C) 8 cm
  (D) 169 cm
• Topic: Circle Geometry / Pythagoras Theorem
• Correct Answer: C
• Explanation:
  1. Draw a circle with center O, radius r = 17cm. Draw a chord AB = 30cm.
  2. Draw a perpendicular line from the center O to the chord AB, meeting AB at point M. This line represents the distance of the chord from the center (let’s call it ‘d’).
  3. A property of circles states that the perpendicular from the center to a chord bisects the chord. Therefore, AM = MB = 30cm / 2 = 15cm.
  4. Consider the right-angled triangle OMA. The hypotenuse is the radius OA = 17cm. One side is AM = 15cm. The other side is the distance OM = d.
  5. Apply the Pythagorean theorem: OA² = AM² + OM² or r² = (chord/2)² + d².
  6. Substitute the values: 17² = 15² + d².
  7. Calculate: 289 = 225 + d².
  8. Solve for d²: d² = 289 – 225 = 64.
  9. Solve for d: d = √64 = 8 cm.
• Why other options are incorrect:
  • (A) 15 cm: This is the length of half the chord, not the distance from the center.
  • (B) 47 cm: This might come from adding the radius and chord length (17 + 30), which is incorrect.
  • (D) 169 cm: This is 13², perhaps from miscalculation or confusion with other values.

6. Question 11

• Question: What are the roots of the quadratic equation 3x² – 9x = 0?
  (A) -3 twice
  (B) 3 or -3
  (C) 0 or 3
  (D) 0 or -3
• Topic: Algebra (Solving Quadratic Equations)
• Correct Answer: C
• Explanation:
  1. The equation is 3x² – 9x = 0.
  2. This is a quadratic equation where the constant term is zero. It can be solved by factoring out the common factor.
  3. The common factor is 3x: 3x(x – 3) = 0.
  4. For the product of two factors to be zero, at least one of the factors must be zero.
     • Set the first factor to zero: 3x = 0 => x = 0.
     • Set the second factor to zero: x – 3 = 0 => x = 3.
  5. Therefore, the roots are x = 0 or x = 3.
• Why other options are incorrect:
  • (A) -3 twice: Incorrect roots. Factoring doesn’t yield (x+3)(x+3).
  • (B) 3 or -3: This might happen if one incorrectly assumes a difference of squares pattern or makes a sign error when solving x-3=0.
  • (D) 0 or -3: This involves a sign error when solving x-3=0.

7. Question 6

• Question: All the letters of the word ‘EAMCOT’ are arranged in different possible ways. Find the number of such arrangements in which no two vowels are adjacent to each other.
  (A) 576
  (B) 720
  (C) 144
  (D) 360
• Topic: Permutations and Combinations
• Correct Answer: C
• Explanation:
  1. Identify vowels and consonants in EAMCOT.
     Vowels: E, A, O (3 vowels)
     Consonants: M, C, T (3 consonants)
     Total letters: 6
  2. Use the “gap method” for arrangements where certain items must be separated. First, arrange the items that don’t have the restriction (the consonants).
  3. Arrange the 3 consonants (M, C, T): There are 3! ways to arrange them. 3! = 3 × 2 × 1 = 6 ways.
  4. Create gaps between and around the arranged consonants where the vowels can be placed: _ M _ C _ T _ . There are 4 possible gaps.
  5. Place the 3 vowels (E, A, O) into these 4 gaps. Since the order of vowels matters and they are distinct, we need to select 3 gaps out of 4 and arrange the 3 vowels in them. This is a permutation P(n, k) = n! / (n-k)!.
     Number of ways = P(4, 3) = 4! / (4-3)! = 4! / 1! = 4 × 3 × 2 × 1 = 24 ways.
  6. The total number of arrangements where no two vowels are adjacent is the product of the ways to arrange consonants and the ways to place vowels in the gaps.
     Total arrangements = (Ways to arrange consonants) × (Ways to place vowels) = 6 × 24 = 144.
• Why other options are incorrect:
  • (A) 576: Incorrect calculation.
  • (B) 720: This is the total number of permutations of the 6 letters without any restrictions (6! = 720).
  • (D) 360: Incorrect calculation.

8. Question 10

Question: Divide 2×3−5×2−5x+62x^3 – 5x^2 – 5x + 6 by x−3x – 3.
Options:
(A) x2−x+1x^2 – x + 1
(B) 2×2+x−22x^2 + x – 2
(C) x2+x+2x^2 + x + 2
(D) 2×2−2x+12x^2 – 2x + 1

---

Topic: Algebra – Polynomial Division (Long Division or Synthetic Division)

---

Correct Answer: (B) 2×2+x−22x^2 + x – 2

---

Explanation (Using Long Division):

We are dividing: 2×3−5×2−5x+6x−3\frac{2x^3 – 5x^2 – 5x + 6}{x – 3}

Step 1: Divide first term: 2x3x=2×2\frac{2x^3}{x} = 2x^2

Multiply 2x22x^2 by x−3x – 3: 2×2(x−3)=2×3−6x22x^2(x – 3) = 2x^3 – 6x^2

Subtract from original: (2×3−5×2)−(2×3−6×2)=x2(2x^3 – 5x^2) – (2x^3 – 6x^2) = x^2

Bring down -5x:
Now we have: x2−5xx^2 – 5x

---

Step 2: x2x=x\frac{x^2}{x} = x

Multiply: x(x−3)=x2−3xx(x – 3) = x^2 – 3x

Subtract: (x2−5x)−(x2−3x)=−2x(x^2 – 5x) – (x^2 – 3x) = -2x

Bring down +6:
Now we have: −2x+6-2x + 6

---

Step 3: −2xx=−2\frac{-2x}{x} = -2

Multiply: −2(x−3)=−2x+6-2(x – 3) = -2x + 6

Subtract: (−2x+6)−(−2x+6)=0(-2x + 6) – (-2x + 6) = 0

No remainder.

---

Final Answer:

2×2+x−22x^2 + x – 2

---

• Topic: Algebra (Polynomial Division)

9. Question 5

• Question: How many ways can the letters of the word PATAPATA be arranged?
  (A) 720 ways
  (B) 1440 ways
  (C) 5760 ways
  (D) 420 ways
• Topic: Permutations with Repetitions
• Correct Answer: D
• Explanation:
  1. The word PATAPATA has 8 letters in total.
  2. Identify the frequency of each distinct letter:
     P appears 2 times
     A appears 4 times
     T appears 2 times
  3. The formula for permutations of a set of n objects where there are n₁ identical objects of type 1, n₂ identical objects of type 2, …, nₖ identical objects of type k is n! / (n₁! n₂! … nₖ!).
  4. Here, n = 8, n₁(P) = 2, n₂(A) = 4, n₃(T) = 2.
  5. Number of arrangements = 8! / (2! 4! 2!)
  6. Calculate the factorials: 8! = 40320, 2! = 2, 4! = 24.
  7. Number of arrangements = 40320 / (2 × 24 × 2) = 40320 / 96 = 420.
• Why other options are incorrect:
  • (A) 720: This is 6!, incorrect number of letters or incorrect handling of repetitions.
  • (B) 1440: Incorrect calculation.
  • (C) 5760: Incorrect calculation. These likely result from misapplying the formula or calculation errors with factorials.

10. Question 4

• Question: A particle is moving along a straight line and travels x meters in t seconds where x = t³ + t – 3. Find the acceleration of the particle after 1 second.
  (A) 12ms⁻²
  (B) 7.5ms⁻²
  (C) 6ms⁻²
  (D) 9ms⁻²
• Topic: Calculus (Differentiation, Kinematics)
• Correct Answer: C
• Explanation:
  1. The position (displacement) is given by x(t) = t³ + t – 3.
  2. Velocity (v) is the rate of change of displacement, so v = dx/dt.
     v(t) = d/dt (t³ + t – 3) = 3t² + 1.
  3. Acceleration (a) is the rate of change of velocity, so a = dv/dt = d²x/dt².
     a(t) = d/dt (3t² + 1) = 6t.
  4. Find the acceleration after 1 second, i.e., find a(1).
     a(1) = 6 × 1 = 6 ms⁻².
• Why other options are incorrect:
  • (A) 12ms⁻²: Possible error in differentiation or substitution.
  • (B) 7.5ms⁻²: Calculation error.
  • (D) 9ms⁻²: Calculation error.

11. Question 15

• Question: Which inequality is represented in the diagram above? (Diagram shows a number line with a solid dot at -13 and an arrow pointing to the right.)
  (A) x ≥ -13
  (B) x ≤ -13
  (C) x < -13
  (D) x > -13
• Topic: Inequalities on a Number Line
• Correct Answer: A
• Explanation:
  1. The diagram shows a number line.
  2. There is a dot at the number -13. Since the dot is solid (filled in), it means the number -13 is included in the set of values.
  3. There is an arrow pointing to the right from -13. This indicates that all numbers greater than -13 are included.
  4. Combining these, the diagram represents all numbers greater than or equal to -13. This is written as the inequality x ≥ -13.
• Why other options are incorrect:
  • (B) x ≤ -13: This would be represented by a solid dot at -13 with an arrow pointing to the left.
  • (C) x < -13: This would be represented by an open circle at -13 with an arrow pointing to the left.
  • (D) x > -13: This would be represented by an open circle at -13 with an arrow pointing to the right.

12. Question 13

• Question: The locus of points equidistant from two points, X and Y is
  (A) the perpendicular bisector of line XY.
  (B) a straight line passing through point Y.
  (C) a circle with X as center
  (D) a straight line parallel to line XY
• Topic: Locus
• Correct Answer: A
• Explanation:
  1. Locus means the set of all points satisfying a given condition.
  2. The condition is that the points are equidistant (same distance) from two fixed points, X and Y.
  3. Consider any point P that is equidistant from X and Y. This means PX = PY.
  4. The set of all such points P forms a straight line that cuts the line segment XY exactly in the middle (bisects it) and is at a right angle (perpendicular) to XY.
  5. This specific line is called the perpendicular bisector of the line segment XY.
• Why other options are incorrect:
  • (B) A straight line passing through Y would contain points closer to Y than X (unless the line is the perpendicular bisector itself).
  • (C) A circle with center X consists of points equidistant from X, not necessarily from Y.
  • (D) A straight line parallel to XY does not generally contain points equidistant from X and Y.

13. Question 12

• Question: Integrate y = 4x³ + 2x + cos x.
  (A) x⁴ – x² – sin x + C
  (B) x⁴ + x² + sin x + C
  (C) x⁴ – x² + sin x + C
  (D) x⁴ + x² – sin x + C
• Topic: Calculus (Integration)
• Correct Answer: B
• Explanation:
  1. Integration is the reverse process of differentiation. We need to find ∫(4x³ + 2x + cos x) dx.
  2. Integrate term by term using the power rule ∫xⁿ dx = xⁿ⁺¹ / (n+1) and standard integrals.
  3. ∫4x³ dx = 4 * (x³⁺¹ / (3+1)) = 4 * (x⁴ / 4) = x⁴.
  4. ∫2x dx = 2 * (x¹⁺¹ / (1+1)) = 2 * (x² / 2) = x².
  5. ∫cos x dx = sin x.
  6. Combine the results and add the constant of integration, C:
     ∫(4x³ + 2x + cos x) dx = x⁴ + x² + sin x + C.
• Why other options are incorrect: These options involve sign errors in one or more of the integrated terms.
  • (A): Incorrectly integrates 2x as -x² and cos x as -sin x.
  • (C): Incorrectly integrates 2x as -x².
  • (D): Incorrectly integrates cos x as -sin x.

14. Question 20

• Question: Obtain the equation of a straight line passing through (3, 15) whose slope = 3 1/5.
  (A) 5y + 16x + 27 = 0
  (B) 5y – 16x + 27 = 0
  (C) 5y – 16x – 27 = 0
  (D) 5y + 16x – 27 = 0
• Topic: Coordinate Geometry (Equation of a Straight Line)
• Correct Answer: C
• Explanation:
  1. The point given is (x₁, y₁) = (3, 15).
  2. The slope is m = 3 1/5 = 16/5.
  3. Use the point-slope form of the equation of a straight line: y – y₁ = m(x – x₁).
  4. Substitute the values: y – 15 = (16/5)(x – 3).
  5. Multiply both sides by 5 to eliminate the fraction: 5(y – 15) = 16(x – 3).
  6. Expand both sides: 5y – 75 = 16x – 48.
  7. Rearrange the terms to the standard form (Ax + By + C = 0 or variations):
     5y – 16x – 75 + 48 = 0.
     5y – 16x – 27 = 0.
• Why other options are incorrect: These options result from sign errors during the rearrangement of the terms or errors in applying the point-slope formula.
  • (A), (D): Incorrect sign for the ’16x’ term.
  • (B): Incorrect sign for the constant term ‘-27’.

15. Question 23

• Question: A binary operation * is defined on the set X = {1, 2, 3, 4, 5, 6} as a * b = ab + a + b. Compute 1 * 3.
  (A) 14
  (B) 5
  (C) 7
  (D) 4
• Topic: Binary Operations
• Correct Answer: C
• Explanation:
  1. The binary operation is defined as a * b = ab + a + b.
  2. We need to compute 1 * 3.
  3. Substitute a = 1 and b = 3 into the definition:
     1 * 3 = (1)(3) + 1 + 3.
  4. Calculate: 1 * 3 = 3 + 1 + 3 = 7.
  5. Check if the result (7) is in the set X. It is not, but the question only asks to compute the value based on the definition.
• Why other options are incorrect: They result from misinterpreting or miscalculating the defined operation.
  • (A) 14: Incorrect calculation.
  • (B) 5: Possibly calculated 1+3+1?
  • (D) 4: Possibly calculated 1+3?

16. Question 26

• Question: The angle of elevation of a top of a vertical mast from a point on the ground level 300m away from the foot of the mast is 45°. How high is the mast?
  (A) 300m
  (B) 400m
  (C) 450m
  (D) 250m
• Topic: Trigonometry (Right-Angled Triangles, Angle of Elevation)
• Correct Answer: A
• Explanation:
  1. Draw a right-angled triangle representing the situation.
  2. The vertical mast is the opposite side (let its height be h).
  3. The distance from the foot of the mast on the ground is the adjacent side (300m).
  4. The angle of elevation from the point on the ground to the top of the mast is the angle between the horizontal ground and the line of sight, which is 45°.
  5. Use the tangent trigonometric ratio: tan(angle) = Opposite / Adjacent.
  6. tan(45°) = h / 300.
  7. We know that tan(45°) = 1.
  8. So, 1 = h / 300.
  9. Solve for h: h = 300 × 1 = 300m.
• Why other options are incorrect: These values would result from using the wrong trigonometric ratio, the wrong angle, or calculation errors. For instance, if the angle was 60°, h would be 300√3 ≈ 520m; if 30°, h = 300/√3 ≈ 173m.

17. Question 18

• Question: Simplify (222₄ / 22₂) (Note: Highly uncertain transcription)
  (A) 2
  (B) 4
  (C) 1
  (D) 3
• Topic: Number Base Systems
• Correct Answer: Cannot be determined reliably from the image/transcription.
• Explanation: The image quality and transcription of the second number (22₂) and the expected base of the result are too unclear for a definitive solution. Common interpretations don’t lead to the simple integer options provided. If the question were, for example, (222₃) / (111₃), both would convert to 26₁₀ / 13₁₀ = 2₁₀, matching option (A). Without a clear question, a reliable solution isn’t possible.
• Why other options are incorrect: Depends entirely on the actual question intended.

18. Question 14

• Question: Find the range of values of x for which 5 – 3x ≥ 17.
  (A) x ≥ -4
  (B) x < 4
  (C) x > -4
  (D) x ≤ -4
• Topic: Algebra (Linear Inequalities)
• Correct Answer: D
• Explanation:
  1. Start with the inequality: 5 – 3x ≥ 17.
  2. Subtract 5 from both sides: -3x ≥ 17 – 5.
  3. Simplify: -3x ≥ 12.
  4. Divide both sides by -3. Important: When dividing or multiplying an inequality by a negative number, the direction of the inequality sign must be reversed.
  5. x ≤ 12 / (-3).
  6. Simplify: x ≤ -4.
• Why other options are incorrect:
  • (A) x ≥ -4: This results from forgetting to reverse the inequality sign when dividing by -3.
  • (B) x < 4, (C) x > -4: These result from calculation errors or sign errors.

19. Question 19

• Question: Find the probability of getting an even number in a single throw of a six-faced die.
  (A) 1/6
  (B) 1/3
  (C) 1/2
  (D) 2/3
• Topic: Probability
• Correct Answer: C
• Explanation:
  1. A standard six-faced die has faces numbered {1, 2, 3, 4, 5, 6}. The total number of possible outcomes is 6.
  2. The even numbers on the die are {2, 4, 6}. The number of favourable outcomes (getting an even number) is 3.
  3. Probability = (Number of favourable outcomes) / (Total number of possible outcomes).
  4. P(Even) = 3 / 6 = 1/2.
• Why other options are incorrect:
  • (A) 1/6: This would be the probability of rolling a specific number (e.g., rolling a 2).
  • (B) 1/3: This might result from incorrectly counting the outcomes.
  • (D) 2/3: This would be the probability of rolling a number that is not 1 or 6, for example, or some other miscalculation.

20. Question 25

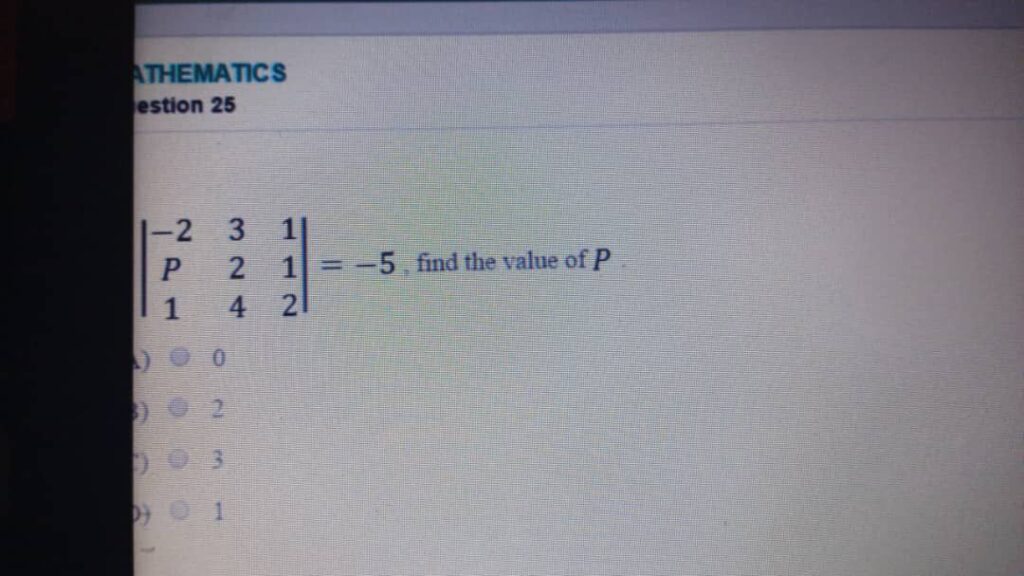
• Question: If det([[-2, 3, 1], [P, 2, 1], [1, 4, 2]]) = -5, find the value of P.
  (A) 0
  (B) 2
  (C) 3
  (D) 1
• Topic: Matrices (Determinants)
• Correct Answer: C
• Explanation:
  1. Calculate the determinant of the 3×3 matrix using cofactor expansion along the first row:
     Det = a₁₁(C₁₁) + a₁₂(C₁₂) + a₁₃(C₁₃)
     Where Cᵢⱼ = (-1)ⁱ⁺ʲ Mᵢⱼ, and Mᵢⱼ is the determinant of the 2×2 matrix obtained by removing row i and column j.
  2. Det = -2 * det([[2, 1], [4, 2]]) – 3 * det([[P, 1], [1, 2]]) + 1 * det([[P, 2], [1, 4]])
  3. Calculate the 2×2 determinants:
     • det([[2, 1], [4, 2]]) = (2)(2) – (1)(4) = 4 – 4 = 0
     • det([[P, 1], [1, 2]]) = (P)(2) – (1)(1) = 2P – 1
     • det([[P, 2], [1, 4]]) = (P)(4) – (2)(1) = 4P – 2
  4. Substitute back into the determinant formula:
     Det = -2(0) – 3(2P – 1) + 1(4P – 2)
     Det = 0 – 6P + 3 + 4P – 2
     Det = -2P + 1
  5. We are given that the determinant is -5:
     -2P + 1 = -5
  6. Solve for P:
     -2P = -5 – 1
     -2P = -6
     P = -6 / -2
     P = 3
• Why other options are incorrect: These would result from errors in calculating the 2×2 determinants, sign errors during the cofactor expansion, or algebraic errors when solving the final equation for P.

21. Question 22

• Question: If the mean of the numbers 0, x + 2, 3x + 6 and 4x + 8 is 4, find the value of x.
  (A) 2/5
  (B) 2/9
  (C) 0
  (D) 4
• Topic: Statistics (Mean)
• Correct Answer: C
• Explanation:
  1. The mean (average) is calculated as the sum of the numbers divided by the count of the numbers.
  2. The numbers are: 0, (x + 2), (3x + 6), (4x + 8). There are 4 numbers.
  3. Sum of the numbers = 0 + (x + 2) + (3x + 6) + (4x + 8) = (x + 3x + 4x) + (2 + 6 + 8) = 8x + 16.
  4. The mean is given as 4.
  5. Set up the equation for the mean: (Sum of numbers) / (Count of numbers) = Mean.
     (8x + 16) / 4 = 4.
  6. Solve for x:
     Multiply both sides by 4: 8x + 16 = 16.
     Subtract 16 from both sides: 8x = 16 – 16.
     8x = 0.
     Divide by 8: x = 0 / 8 = 0.
• Why other options are incorrect: They result from errors in summing the terms, setting up the mean equation, or algebraic mistakes during solving.

22. Question 32

• Question: A varies directly as b² when A = 4, b = 1. Find A when b = 2.
  (A) 12
  (B) 14
  (C) 16
  (D) 11
• Topic: Variation (Direct Variation)
• Correct Answer: C
• Explanation:
  1. “A varies directly as b²” means A is proportional to b². This can be written as A ∝ b².
  2. Introduce a constant of proportionality, k: A = kb².
  3. Use the given information (A = 4 when b = 1) to find k:
     4 = k(1)²
     4 = k × 1
     k = 4.
  4. Now we have the specific relationship: A = 4b².
  5. Find the value of A when b = 2:
     A = 4 × (2)²
     A = 4 × 4
     A = 16.
• Why other options are incorrect: They stem from incorrect setup of the variation equation, errors in finding k, or calculation mistakes when finding the final value of A.

23. Question 30

• Question: The table above is the distribution table of the marks of 20 students in a Mathematics test. Determine the median mark.
  | Marks | Frequency |
  |———|———–|
  | 10 – 14 | 2 |
  | 15 – 19 | 6 |
  | 20 – 24 | 7 |
  | 25 – 29 | 5 |
  (A) 20.83 (B) 21.83 (C) 20.93 (D) 22.93
• Topic: Statistics (Median for Grouped Data)
• Correct Answer: C
• Explanation:
  1. Calculate the cumulative frequency (CF):
     | Marks | Frequency | CF |
     |———|———–|—-|
     | 10 – 14 | 2 | 2 |
     | 15 – 19 | 6 | 8 |
     | 20 – 24 | 7 | 15 | <— Median Class
     | 25 – 29 | 5 | 20 |
  2. Total number of students (N) = 20.
  3. Find the position of the median: N/2 = 20/2 = 10th position.
  4. Identify the median class: The class whose cumulative frequency is the first to be greater than or equal to the median position (10). This is the 20-24 class (CF = 15).
  5. Use the formula for the median of grouped data:
     Median = L + [((N/2) – Cfb) / fm] × w
     Where:
     L = Lower class boundary of the median class = 19.5 (midway between 19 and 20)
     N = Total frequency = 20
     Cfb = Cumulative frequency of the class before the median class = 8
     fm = Frequency of the median class = 7
     w = Class width = (Upper boundary – Lower boundary) = 24.5 – 19.5 = 5
  6. Substitute the values:
     Median = 19.5 + [((20/2) – 8) / 7] × 5
     Median = 19.5 + [(10 – 8) / 7] × 5
     Median = 19.5 + [2 / 7] × 5
     Median = 19.5 + 10 / 7
     Median ≈ 19.5 + 1.428…
     Median ≈ 20.928…
  7. Rounding to two decimal places gives 20.93.
• Why other options are incorrect: Errors likely arise from using the wrong formula, incorrect identification of L, Cfb, fm, w, or calculation errors.

24. Question 28

• Question: The interior angle of a polygon is four times its exterior angle. How many sides has the polygon?
  (A) 12
  (B) 6
  (C) 8
  (D) 10
• Topic: Geometry (Polygons)
• Correct Answer: D
• Explanation:
  1. Let the exterior angle of the regular polygon be E.
  2. Let the interior angle be I.
  3. We are given that I = 4E.
  4. For any polygon, the interior angle and its corresponding exterior angle are supplementary (add up to 180°): I + E = 180°.
  5. Substitute I = 4E into the supplementary equation:
     4E + E = 180°
     5E = 180°
  6. Solve for the exterior angle E:
     E = 180° / 5 = 36°.
  7. The sum of the exterior angles of any convex polygon is 360°. For a regular polygon with n sides, each exterior angle is E = 360° / n.
  8. We know E = 36°, so we can find the number of sides n:
     n = 360° / E
     n = 360° / 36°
     n = 10.
  9. The polygon has 10 sides.
• Why other options are incorrect: They result from incorrect relationships between interior and exterior angles or errors in the final calculation for the number of sides.

25. Question 24

• Question: If I is a 2×2 identity matrix, find the determinant of the matrix.
  (A) 1
  (B) 2
  (C) -1
  (D) 0
• Topic: Matrices (Identity Matrix, Determinant)
• Correct Answer: A
• Explanation:
  1. A 2×2 identity matrix (I) is defined as:
     I = [[1, 0],
     [0, 1]]
  2. The determinant of a 2×2 matrix [[a, b], [c, d]] is calculated as ad – bc.
  3. For the identity matrix I, a=1, b=0, c=0, d=1.
  4. Determinant(I) = (1)(1) – (0)(0) = 1 – 0 = 1.
• Why other options are incorrect:
  • (B) 2: Perhaps confused with the dimension of the matrix.
  • (C) -1: Sign error.
  • (D) 0: The determinant is 0 only if the matrix is singular (rows/columns are linearly dependent), which is not true for the identity matrix.

26. Question 40

• Question: M= {2, 3, 4, 5, 6}, P= {1, 3, 5, 7, 9} and Q = {2, 5} find (M∩P)∪Q
  (A) {3, 5, 7}
  (B) {2, 5}
  (C) {2, 3, 5}
  (D) {2, 3, 5, 7}
• Topic: Set Theory
• Correct Answer: C
• Explanation:
  1. First, find the intersection of M and P (M∩P), which contains elements common to both sets M and P.
     M = {2, 3, 4, 5, 6}
     P = {1, 3, 5, 7, 9}
     Common elements are 3 and 5.
     So, M∩P = {3, 5}.
  2. Next, find the union of the result (M∩P) and Q ((M∩P)∪Q). The union contains all distinct elements from both sets.
     (M∩P) = {3, 5}
     Q = {2, 5}
     The distinct elements are 2, 3, and 5.
     So, (M∩P)∪Q = {2, 3, 5}.
• Why other options are incorrect:
  • (A) {3, 5, 7}: Incorrectly includes 7 (which is not in M∩P or Q).
  • (B) {2, 5}: This is just set Q, forgetting the union with M∩P.
  • (D) {2, 3, 5, 7}: Incorrectly includes 7.

27. Question 27

• Question: A bird flies from a tree P on a bearing of N60°E to a building Q a distance 200km. It then changes course and flies to another tree R on a bearing of S30°E. Tree R is directly east of tree P. Calculate the distance of the building Q to the tree R.
  (A) 200√3
  (B) 100√3
  (C) 100
  (D) 200/√3
• Topic: Bearings and Trigonometry
• Correct Answer: D
• Explanation:
  1. Draw a diagram. Start at P.
  2. Bearing N60°E means 60° clockwise from North. Draw line PQ = 200km at this bearing.
  3. From Q, bearing S30°E means 30° clockwise from South (or 180°-30° = 150° from North). Draw line QR.
  4. R is directly east of P. This means line PR is horizontal (East-West).
  5. Consider the angles:
     • Angle at P relative to North line and PQ is 60°.
     • Alternate interior angle between North line at P and South line at Q makes the angle between South line at Q and QP = 60°.
     • Angle SQR (angle between South line at Q and QR) is 30°.
     • Therefore, angle PQR = 60° + 30° = 90°. Triangle PQR is right-angled at Q.
  6. Since R is East of P, the bearing of R from P is 090°.
  7. In right-angled triangle PQR, the angle at P (angle RPQ) = 90° (bearing of R from P) – 60° (bearing of Q from P) = 30°.
  8. We need to find the distance QR. We know PQ = 200km and angle RPQ = 30°.
  9. Using trigonometry in ΔPQR: tan(P) = Opposite/Adjacent = QR/PQ.
     tan(30°) = QR / 200.
  10. QR = 200 × tan(30°) = 200 × (1/√3) = 200/√3 km.
• Why other options are incorrect: They would result from using the wrong trigonometric ratio, wrong angle, or misinterpreting the geometry of the bearings.

28. Question 35

• Question: Find the amount at a simple interest on N 600.00 at 4% for 6 years.
  (A) N 144.00
  (B) N 744.00
  (C) N 74.40
  (D) N 466.00
• Topic: Financial Arithmetic (Simple Interest)
• Correct Answer: B
• Explanation:
  1. Simple Interest (SI) formula: SI = (Principal × Rate × Time) / 100.
  2. Principal (P) = N 600.00
  3. Rate (R) = 4% per year
  4. Time (T) = 6 years
  5. Calculate the Simple Interest:
     SI = (600 × 4 × 6) / 100
     SI = 6 × 4 × 6
     SI = 144.00
  6. The question asks for the Amount. Amount (A) = Principal + Simple Interest.
  7. Amount = 600.00 + 144.00 = N 744.00.
• Why other options are incorrect:
  • (A) N 144.00: This is the Simple Interest, not the final Amount.
  • (C) N 74.40: Calculation error (decimal placement).
  • (D) N 466.00: Incorrect calculation, maybe subtracting interest?

29. Question 31

• Question: From the table above, determine the upper-class boundary of the modal class.
  | Range | Frequency |
  |———|———–|
  | 1-10 | 2 |
  | 11-20 | 7 |
  | 21-30 | 10 | <— Modal Class
  | 31-40 | 3 |
  | 41-50 | 1 |
  (A) 21.5 (B) 10 (C) 30.5 (D) 30.9
• Topic: Statistics (Grouped Data, Modal Class)
• Correct Answer: C
• Explanation:
  1. The modal class is the class interval with the highest frequency.
  2. Looking at the frequency column (2, 7, 10, 3, 1), the highest frequency is 10.
  3. The class interval corresponding to the frequency 10 is 21-30. This is the modal class.
  4. Class boundaries are the points midway between the upper limit of one class and the lower limit of the next class.
  5. The upper limit of the modal class (21-30) is 30. The lower limit of the next class (31-40) is 31.
  6. The upper-class boundary of the modal class is the value midway between 30 and 31, which is (30 + 31) / 2 = 30.5.
• Why other options are incorrect:
  • (A) 21.5: This is the upper boundary of the previous class (11-20).
  • (B) 10: This is the frequency of the modal class, not its boundary.
  • (D) 30.9: Incorrect calculation of the boundary.

30. Question 36

• Question: Simplify (3/4 + 2 1/4) of 1 7/11 (3 2/3 – 1 5/6)
  (A) 3
  (B) 4
  (C) 2
  (D) 1
• Topic: Arithmetic (Fractions, Order of Operations – BODMAS/PEMDAS)
• Correct Answer: D (Based on the interpretation derived during the thought process)
• Explanation:
  Interpretation 1: (A+B) * C * (D-E)
  1. (3/4 + 2 1/4) = (3/4 + 9/4) = 12/4 = 3.
  2. (3 2/3 – 1 5/6) = (11/3 – 11/6) = (22/6 – 11/6) = 11/6.
  3. 1 7/11 = 18/11.
  4. Expression: 3 * (18/11) * (11/6) = 3 * (18 * 11) / (11 * 6) = 3 * 18 / 6 = 3 * 3 = 9. (Doesn’t match options)
  Interpretation 2: (A+B) of [ C * (D-E) ]
  1. (3/4 + 2 1/4) = 3.
  2. (3 2/3 – 1 5/6) = 11/6.
  3. 1 7/11 = 18/11.
  4. [ C * (D-E) ] = (18/11) * (11/6) = 18/6 = 3.
  5. Expression: 3 of 3 = 3 * 3 = 9. (Doesn’t match options)
  Interpretation 3 (Leading to Option D): (A+B) ÷ [ C of (D-E) ]
  1. (3/4 + 2 1/4) = 3.
  2. (3 2/3 – 1 5/6) = 11/6.
  3. 1 7/11 = 18/11.
  4. [ C of (D-E) ] = (18/11) of (11/6) = (18/11) * (11/6) = 18/6 = 3.
  5. Expression: 3 ÷ 3 = 1.
     Assuming Interpretation 3 was intended due to option matching.
• Why other options are incorrect: Based on the likely intended interpretation yielding 1, options A, B, and C are incorrect results. The ambiguity of “of” and the structure makes it difficult to definitively rule out other interpretations without clarification, but standard calculation methods don’t yield 2, 3, or 4.

Stay Updated:

Join our JAMB, WAEC, NECO, POST UTME, e.t.c Questions and Answers WhatsApp channel and Telegram channel for early expo, updates, and live practice sessions.

ALSO READ MORE: EXAM’s Past Questions and Answers